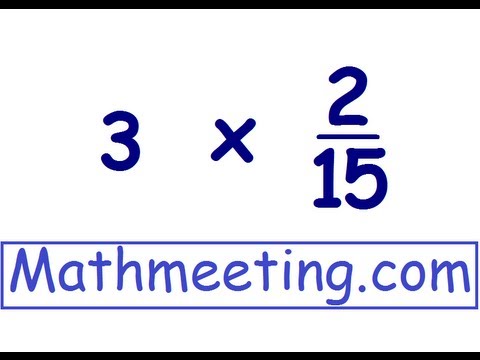
Above is an example of whole number multiplied by a fraction. Here are the steps that I and students can use in order to solve the problem.
Steps:
1. Rewrite the whole number as a fraction. To rewrite a whole number as a fraction, simply place the whole number over 1. (Any number over one is equaled to itself.)
2. Multiply the numerators of the two fractions. (The numerators are the numbers above the line.)
3. Multiply the denominators of the two fractions. (The denominators are the numbers below the line.)
4. Reduce the answer if possible. Your answer most likley will become a proper fraction or an imporper fraction, you have to simplify the answer to its lowest terms. The number in the denominator will have to be divided by the numerator and the denominator to equal your simplified answer.
By using the steps above for the example 3 x 2/15 the answer would be:
3 x 2 = 6 = 2
1 15 15 5
Here is another example of a whole number multiplied by a fraction that is turned into an improper fraction and simplified:

Multiplying whole numbers by fractons can also be shown in models because multiplication is nothing more than repeated addition. So below is a model to show how it multiplyng whole numbers by fractions can be used a a different way:

Though some students might not be comfortable with word problems, they should be able to understand and extract the key details that is needed to solve a word problem. I would provide the students with a fun word problem activity for them to do individually or in pairs.
CCSS.MATH.CONTENT.4.NF.B.4
Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.
Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.
CCSS.MATH.CONTENT.4.NF.B.4.A
Understand a fraction a/b as a multiple of 1/b. For example, use a visual fraction model to represent 5/4 as the product 5 × (1/4), recording the conclusion by the equation 5/4 = 5 × (1/4).
Understand a fraction a/b as a multiple of 1/b. For example, use a visual fraction model to represent 5/4 as the product 5 × (1/4), recording the conclusion by the equation 5/4 = 5 × (1/4).
CCSS.MATH.CONTENT.4.NF.B.4.B
Understand a multiple of a/b as a multiple of 1/b, and use this understanding to multiply a fraction by a whole number. For example, use a visual fraction model to express 3 × (2/5) as 6 × (1/5), recognizing this product as 6/5. (In general, n × (a/b) = (n × a)/b.)
Understand a multiple of a/b as a multiple of 1/b, and use this understanding to multiply a fraction by a whole number. For example, use a visual fraction model to express 3 × (2/5) as 6 × (1/5), recognizing this product as 6/5. (In general, n × (a/b) = (n × a)/b.)
CCSS.MATH.CONTENT.4.NF.B.4.C
Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?
Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?

I love love love this post. I happen to like fractions (most of the time lol) But many people have problems with them, especially when it comes to multiplying and dividing them. To me, that is part of the fun. haha. I also could not agree with you more that word problems needs to be addressed and worked on so students can be comfortable with what they reading and determine what operation they need to be doing.
ReplyDelete